A Regular Polygon Is Equiangular

Example equiangular polygons
Direct	Indirect	Skew
A rectangle, <four>, is a convex direct equiangular polygon, containing four 90° internal angles.	A concave indirect equiangular polygon, <6-2>, like this hexagon, counterclockwise, has v left turns and one right plough, like this tetromino.	A skew polygon has equal angles off a plane, like this skew octagon alternating red and bluish edges on a cube.
Directly	Indirect	Counter-turned
A multi-turning equiangular polygon can be direct, like this octagon, <viii/two>, has 8 ninety° turns, totaling 720°.	A concave indirect equiangular polygon, <5-ii>, counterclockwise has 4 left turns and one right turn. (-1.2.four.iii.two)_60°	An indirect equiangular hexagon, <6-6>_xc° with 3 left turns, 3 right turns, totaling 0°.

In Euclidean geometry, an equiangular polygon is a polygon whose vertex angles are equal. If the lengths of the sides are also equal (that is, if it is too equilateral) then it is a regular polygon. Isogonal polygons are equiangular polygons which alternate ii edge lengths.

For clarity, a planar equiangular polygon tin can exist called direct or indirect. A directly equiangular polygon has all angles turning in the same direction in a airplane and can include multiple turns. Convex equiangular polygons are e'er direct. An indirect equiangular polygon can include angles turning right or left in any combination. A skew equiangular polygon may be isogonal, but can't exist considered directly since it is nonplanar.

A spirolateral n _θ is a special case of an equiangular polygon with a set of n integer edge lengths repeating sequence until returning to the outset, with vertex internal angles θ.

Construction [edit]

An equiangular polygon tin be constructed from a regular polygon or regular star polygon where edges are extended as infinite lines. Each edges can exist independently moved perpendicular to the line's direction. Vertices represent the intersection point between pairs of neighboring line. Each moved line adjusts its edge-length and the lengths of its two neighboring edges.^[1] If edges are reduced to zero length, the polygon becomes degenerate, or if reduced to negative lengths, this will reverse the internal and external angles.

For an even-sided direct equiangular polygon, with internal angles θ°, moving alternate edges can invert all vertices into supplementary angles, 180-θ°. Odd-sided directly equiangular polygons can only be partially inverted, leaving a mixture of supplementary angles.

Every equiangular polygon can be adjusted in proportions by this construction and still preserve equiangular status.

Equiangular polygon theorem [edit]

For a convex equiangular p-gon, each internal angle is 180(1-2/p)°; this is the equiangular polygon theorem.

For a directly equiangular p/q star polygon, density q, each internal bending is 180(ane-2q/p)°, with 1<2q<p. For w=gcd(p,q)>one, this represents a w-wound (p/west)/(q/w) star polygon, which is degenerate for the regular case.

A concave indirect equiangular (p _r+p _fifty)-gon, with p _r right plough vertices and p _l left turn vertices, will take internal angles of 180(one-2/|p _r-p _fifty|))°, regardless of their sequence. An indirect star equiangular (p _r+p _l)-gon, with p _r right turn vertices and p _l left plow vertices and q full turns, will have internal angles of 180(1-2q/|p _r-p ₅₀|))°, regardless of their sequence. An equiangular polygon with the same number of right and left turns has zero total turns, and has no constraints on its angles.

Notation [edit]

Every straight equiangular p-gon can be given a annotation <p> or <p/q>, like regular polygons {p} and regular star polygons {p/q}, containing p vertices, and stars having density q.

Convex equiangular p-gons <p> accept internal angles 180(i-ii/p)°, while directly star equiangular polygons, <p/q>, accept internal angles 180(1-2q/p)°.

A concave indirect equiangular p-gon tin can be given the notation <p-2c>, with c counter-plough vertices. For example, <6-ii> is a hexagon with ninety° internal angles of the departure, <4>, 1 counter-turned vertex. A multiturn indirect equilateral p-gon can be given the notation <^p-twoc/_q> with c counter turn vertices, and q total turns. An equiangular polygon <p-p> is a p-gon with undefined internal angles θ, just tin can be expressed explicitly as <p-p>_θ.

Other properties [edit]

Viviani'south theorem holds for equiangular polygons:^[2]

The sum of distances from an interior point to the sides of an equiangular polygon does non depend on the location of the indicate, and is that polygon's invariant.

A cyclic polygon is equiangular if and only if the alternate sides are equal (that is, sides 1, 3, v, ... are equal and sides 2, iv, ... are equal). Thus if n is odd, a cyclic polygon is equiangular if and only if it is regular.^[3]

For prime p, every integer-sided equiangular p-gon is regular. Moreover, every integer-sided equiangular p ^m-gon has p-fold rotational symmetry.^[4]

An ordered fix of side lengths $(a_{1},\dots ,a_{n})$ gives rising to an equiangular north-gon if and simply if either of two equivalent conditions holds for the polynomial $a_{ane}+a_{2}ten+\cdots +a_{due north-i}x^{northward-2}+a_{north}10^{n-1}:$ it equals zero at the complex value $eastward^{2\pi i/n};$ it is divisible by $10^{2}-2x\cos(two\pi /n)+1.$ ^[v]

Straight equiangular polygons past sides [edit]

Straight equiangular polygons can be regular, isogonal, or lower symmetries. Examples for <p/q> are grouped into sections past p and subgrouped by density q.

Equiangular triangles [edit]

Equiangular triangles must be convex and have 60° internal angles. It is an equilateral triangle and a regular triangle, <3>={3}. The just degree of liberty is edge-length.

Regular, {iii}, r₆

Equiangular quadrilaterals [edit]

A rectangle dissected into a 2×3 assortment of squares^{[half dozen]}

Direct equiangular quadrilaterals have 90° internal angles. The but equiangular quadrilaterals are rectangles, <4>, and squares, {iv}.

An equiangular quadrilateral with integer side lengths may be tiled by unit squares.^[6]

Regular, {4}, r_viii

Equiangular pentagons [edit]

Direct equiangular pentagons, <5> and <5/2>, take 108° and 36° internal angles respectively.

108° internal angle from an equiangular pentagon, <5>

Equiangular pentagons can tin be regular, have bilateral symmetry, or no symmetry.

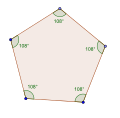
Regular, r₁₀
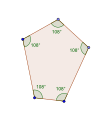
Bilateral symmetry, i₂
No symmetry, a_one

36° internal angles from an equiangular pentagram, <5/2>

Irregular, d₂

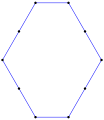
Equiangular hexagons [edit]

An equilateral hexagon with ane:ii edge length ratios, with equilateral triangles.^[6] This is spirolateral 2_120°.

Direct equiangular hexagons, <6> and <6/2>, have 120° and 60° internal angles respectively.

120° internal angles of an equiangular hexagon, <6>

An equiangular hexagon with integer side lengths may be tiled past unit equilateral triangles.^[6]

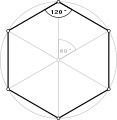
Regular, {six}, r₁₂
Spirolateral (ane…3)_120°, grand₂
Spirolateral (1,two,2)_120°, i₄
Spirolateral (i,2,2,2,i,3)_120°, p_two

60° internal angles of an equiangular double-wound triangle, <6/2>

Regular, degenerate, r₆
Spirolateral (i,two)_60°, p₆
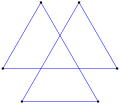
Spirolateral (2,3)_60°, p₆
Spirolateral (ane,ii,three,four,3,2)_60°, p₂

Equiangular heptagons [edit]

Direct equiangular heptagons, <7>, <7/2>, and <7/3> have 128 4/seven°, 77 ane/vii° and 25 5/7° internal angles respectively.

128.57° internal angles of an equiangular heptagon, <7>

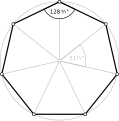
Regular, {seven}, r₁₄
Irregular, i₂

77.14° internal angles of an equiangular heptagram, <7/2>

Regular, r₁₄
Irregular, i₂

25.71° internal angles of an equiangular heptagram, <7/3>

Regular, r_fourteen
Irregular, i₂

Equiangular octagons [edit]

Direct equiangular octagons, <8>, <viii/2> and <8/three>, take 135°, 90° and 45° internal angles respectively.

135° internal angles from an equiangular octagon, <8>

Regular, r₁₆
Spirolateral (1…four)_135°, chiliad₂
Diff truncated square, p_ii

90° internal angles from an equiangular double-wound foursquare, <8/two>

Regular degenerate, r₈
Spirolateral (1,ii,two,3,3,2,2,1)_90°, d₂
Spirolateral (two,ane,3,2,2,3,1,2)_ninety°, d₂

45° internal angles from an equiangular octagram, <eight/3>

Regular, r_xvi
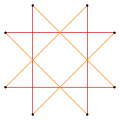
Isogonal, p₈
Isogonal, p_viii
Isogonal, p_eight
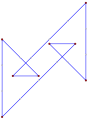
Spirolateral (one…4)_45°, thou₂

Equiangular enneagons [edit]

Direct equiangular enneagons, <9>, <9/2>, <nine/3>, and <nine/4> accept 140°, 100°, 60° and 20° internal angles respectively.

140° internal angles from an equiangular enneagon <ix>

Regular, r₁₈
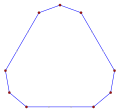
Spirolateral (i,1,3)_140°, i_{half dozen}

100° internal angles from an equiangular enneagram, <9/2>

Regular {9/2}, p₉
Spirolateral (1,1,5)_100°, i₆
Spirolateral three_100°, g₃

60° internal angles from an equiangular triple-wound triangle, <nine/3>

Regular, degenerate, r_vi
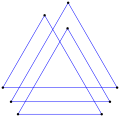
Irregular, a_one
Irregular, a₁
Irregular, a_ane

twenty° internal angles from an equiangular enneagram, <9/4>

Regular {9/4}, r_eighteen
Irregular, i_two

Equiangular decagons [edit]

Direct equiangular decagons, <10>, <10/ii>, <10/3>, <x/four>, take 144°, 108°, 72° and 36° internal angles respectively.

144° internal angles from an equiangular decagon <ten>

Regular, r₂₀
Spirolateral (1…5)_144°, thou_ii

108° internal angles from an equiangular double-wound pentagon <10/2>

Regular, degenerate
Irregular, p₂

72° internal angles from an equiangular decagram <ten/three>

Regular {10/3}, r₂₀
Isogonal, p₁₀
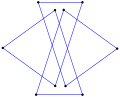
Irregular, i_four
Spirolateral (1…5)_72°, yard₂

36° internal angles from an equiangular double-wound pentagram <10/4>

Regular, degenerate, r₁₀
Isogonal, p₁₀
Isogonal, p₁₀
Irregular, p₂
Irregular, p_two
Irregular, p₂

Equiangular hendecagons [edit]

Direct equiangular hendecagons, <11>, <11/two>, <11/3>, <11/4>, and <xi/5> have 147 3/eleven°, 114 6/eleven°, 81 9/xi°, 49 one/11°, and sixteen 4/xi° internal angles respectively.

147° internal angles from an equiangular hendecagon, <11>

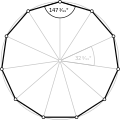
Regular, {11}, r₂₂

114° internal angles from an equiangular hendecagram, <11/2>

Regular {11/2}, r₂₂

81° internal angles from an equiangular hendecagram, <11/3>

Regular {11/three}, r₂₂

49° internal angles from an equiangular hendecagram, <11/4>

Regular {11/4}, r₂₂

16° internal angles from an equiangular hendecagram, <xi/five>

Regular {11/five}, r₂₂

Equiangular dodecagons [edit]

Direct equiangular dodecagons, <12>, <12/2>, <12/3>, <12/4>, and <12/v> accept 150°, 120°, 90°, lx°, and 30° internal angles respectively.

150° internal angles from an equiangular dodecagon, <12>

Convex solutions with integer border lengths may be tiled by pattern blocks, squares, equilateral triangles, and xxx° rhombi.^[6]

Regular, {12}, r₂₄
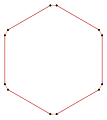
Isogonal, p₁₂
Spirolateral (1…three)_150°, chiliad₄
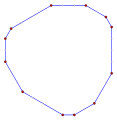
Spirolateral (1…4)_150°, g₃
Spirolateral (ane…six)_150°, k₂

120° internal angles from an equiangular double-wound hexagon, <12/2>

Regular degenerate, r₁₂
Irregular, d_ii
Irregular, d₂

ninety° internal angles from an equiangular triple-wound square, <12/iii>

Regular, degenerate, r₈
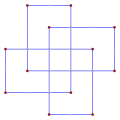
Spirolateral (2…4)_90°, thousand₄
Spirolateral (1,1,3)_90°, i₈
Spirolateral (one,ii,2)_90°, i_viii
Spirolateral (ane…6)_ninety°, g₂
Irregular, a_ane

60° internal angles from an equiangular quadruple-wound triangle, <12/4>

Regular, degenerate, r₆
Spirolateral (one…4)_threescore°, g₃
Irregular, a₁

30° internal angles from an equiangular dodecagram, <12/5>

Regular {12/5}, r₂₄
Isogonal, p₁₂
Spirolateral (1…3)_30°, g_iv
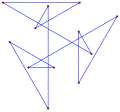
Spirolateral (one…4)_30°, g₃
Spirolateral (1…6)_thirty°, g₂

Equiangular tetradecagons [edit]

Straight equiangular tetradecagons, <xiv>, <14/2>, <fourteen/3>, <14/4>, and <14/5>, <14/6>, have 154 2/7°, 128 4/vii°, 102 6/vii°, 77 one/seven°, 51 3/7° and 25 v/7° internal angles respectively.

154.28° internal angles from an equiangular tetradecagon, <fourteen>

Regular {fourteen}, r₂₈
Isogonal, t{7}, p_xiv

128.57° internal angles from an equiangular double-wound regular heptagon, <xiv/2>

Regular degenerate, r₁₄
Isogonal, t{7/2}, p_xiv

102.85° internal angles from an equiangular tetradecagram, <14/3>

Regular {14/3}, r₂₈
Isogonal t{7/iii}, p₁₄

77.xiv° internal angles from an equiangular double-wound heptagram <14/4>

Regular degenerate, r_xiv
Isogonal, p_fourteen
Isogonal, p₁₄

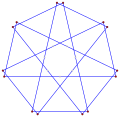
51.43° internal angles from an equiangular tetradecagram, <14/five>

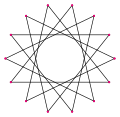
Regular {14/5}, r₂₈
Isogonal, p₁₄
Isogonal, p₁₄

25.71° internal angles from an equiangular double-wound heptagram, <14/6>

Regular degenerate, r_xiv
Isogonal, p_xiv
Isogonal, p₁₄
Isogonal, p₁₄
Irregular, d₂

Equiangular pentadecagons [edit]

Straight equiangular pentadecagons, <15>, <15/two>, <15/3>, <15/4>, <15/5>, <fifteen/6>, and <15/7>, take 156°, 132°, 108°, 84°, sixty° and 12° internal angles respectively.

156° internal angles from an equiangular pentadecagon, <xv>

Regular, {xv}, r₃₀

132° internal angles from an equiangular pentadecagram, <fifteen/2>

Regular, {15/2}, r₃₀

108° internal angles from an equiangular triple-wound pentagon, <15/3>

Regular, degenerate, r₁₀

84° internal angles from an equiangular pentadecagram, <15/four>

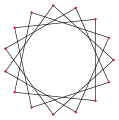
Regular, {15/four}, r_thirty

60° internal angles from an equiangular 5-wound triangle, <15/five>

Regular, degenerate, r_six
Irregular, a₁

36° internal angles from an equiangular triple-wound pentagram, <15/half dozen>

Regular, degenerate, r_x
Irregular, a_ane
Spirolateral (one…4)_36°, g₅

12° internal angles from an equiangular pentadecagram, <xv/7>

Regular, {xv/7}, r₃₀

Equiangular hexadecagons [edit]

Direct equiangular hexadecagons, <xvi>, <16/2>, <16/3>, <16/iv>, <sixteen/5>, <16/vi>, and <sixteen/7>, have 157.5°, 135°, 112.5°, 90°, 67.5° 45° and 22.5° internal angles respectively.

157.five° internal angles from an equiangular hexadecagon, <xvi>

Regular, {xvi}, r₃₂
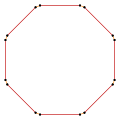
Isogonal, t{8}, p₁₆

135° internal angles from an equiangular double-wound octagon, <16/two>

Regular, degenerate, r_sixteen
Irregular, p₁₆

112.5° internal angles from an equiangular hexadecagram, <16/iii>

Regular, {sixteen/3}, r₃₂

90° internal angles from an equiangular four-wound square, <16/4>

Regular, degenerate, r_viii
Irregular, a₁

67.5° internal angles from an equiangular hexadecagram, <16/5>

Regular, {xvi/5}, r₃₂

45° internal angles from an equiangular double-wound regular octagram, <sixteen/half-dozen>

Regular, degenerate, r₁₆

22.v° internal angles from an equiangular hexadecagram, <xvi/7>

Regular, {16/vii}, r₃₂
Isogonal, p₁₆

Equiangular octadecagons [edit]

Straight equiangular octadecagons, <18}, <18/2>, <xviii/3>, <eighteen/4>, <xviii/5>, <18/6>, <18/7>, and <xviii/8>, have 160°, 140°, 120°, 100°, 80°, 60°, 40° and 20° internal angles respectively.

160° internal angles from an equiangular octadecagon, <18>

Regular, {18}, r₃₆
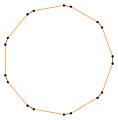
Isogonal, t{ix}, p₁₈

140° internal angles from an equiangular double-wound enneagon, <18/2>

Regular, degenerate

120° internal angles of an equiangular three-wound hexagon <18/3>

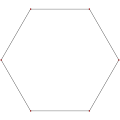
Regular, degenerate, r_eighteen
irregular, a₁

100° internal angles of an equiangular double-wound enneagram <eighteen/4>

Regular, degenerate, r₁₈

80° internal angles of an equiangular octadecagram {xviii/five}

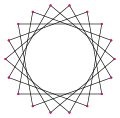
Regular, {18/5}, r₃₆

60° internal angles of an equiangular 6-wound triangle <eighteen/6>

Regular, degenerate, r_six
irregular, a₁

40° internal angles of an equiangular octadecagram <xviii/7>

Regular, {18/7}, r₃₆
Isogonal, p₁₈
Isogonal, p₁₈
Isogonal, p₁₈

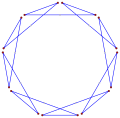
20° internal angles of an equiangular double-wound enneagram <18/8>

Regular, degenerate, r_eighteen
Isogonal, p_eighteen
Isogonal, p₁₈
Isogonal, p₁₈
Isogonal, p₁₈
Spirolateral 6_20°, g₃

Equiangular icosagons [edit]

Direct equiangular icosagon, <20>, <xx/3>, <20/4>, <20/5>, <twenty/6>, <20/vii>, and <xx/9>, have 162°, 126°, 108°, ninety°, 72°, 54° and xviii° internal angles respectively.

162° internal angles from an equiangular icosagon, <xx>

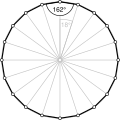
Regular, {xx}, r₄₀

144° internal angles from an equiangular double-wound decagon, <twenty/two>

Regular, degenerate, r_xx

126° internal angles from an equiangular icosagram, <xx/3>

Regular {twenty/3}, p_twoscore

108° internal angles from an equiangular 4-wound pentagon, <twenty/four>

Regular degenerate, r₁₀
Irregular, a₁

90° internal angles from an equiangular 5-wound square, <20/5>

Regular degenerate, r₈

72° internal angles from an equiangular double-wound decagram, <20/half dozen>

Regular degenerate, r₂₀
Spirolateral (1…4)_72°, g₅

54° internal angles from an equiangular icosagram, <twenty/vii>

Regular {xx/7}, r_xl
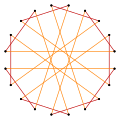
Isogonal, p₂₀
Isogonal, p_twenty
Isogonal, p₂₀

36° internal angles from an equiangular quadruple-wound pentagram, <20/8>

Regular degenerate, r_x
irregular, a₁

18° internal angles from an equiangular icosagram, <20/nine>

Regular {20/9}, r_xl
Isogonal, p₂₀
Isogonal, p_twenty
Isogonal, p₂₀
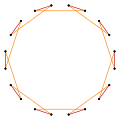
Isogonal, p_twenty

Encounter also [edit]

Spirolateral

References [edit]

^ Marius Munteanu, Laura Munteanu, Rational Equiangular Polygons Applied Mathematics, Vol.4 No.10, October 2013
^ Elias Abboud "On Viviani's Theorem and its Extensions" pp. ii, 11
^ De Villiers, Michael, "Equiangular cyclic and equilateral circumscribed polygons", Mathematical Gazette 95, March 2011, 102-107.
^ McLean, G. Robin. "A powerful algebraic tool for equiangular polygons", Mathematical Gazette 88, November 2004, 513-514.
^ M. Bras-Amorós, One thousand. Pujol: "Side Lengths of Equiangular Polygons (as seen by a coding theorist)", The American Mathematical Monthly, vol. 122, n. five, pp. 476–478, May 2015. ISSN 0002-9890.
^ ^a ^b ^c ^d ^eastward Ball, Derek (2002), "Equiangular polygons", The Mathematical Gazette, 86 (507): 396–407, doi:ten.2307/3621131, JSTOR 3621131, S2CID 233358516 .

Williams, R. The Geometrical Foundation of Natural Structure: A Source Volume of Pattern. New York: Dover Publications, 1979. p. 32

External links [edit]

A Property of Equiangular Polygons: What Is It Nigh? a discussion of Viviani'southward theorem at Cutting-the-knot.
Weisstein, Eric West. "Equiangular Polygon". MathWorld.